Noise with Power-law trends

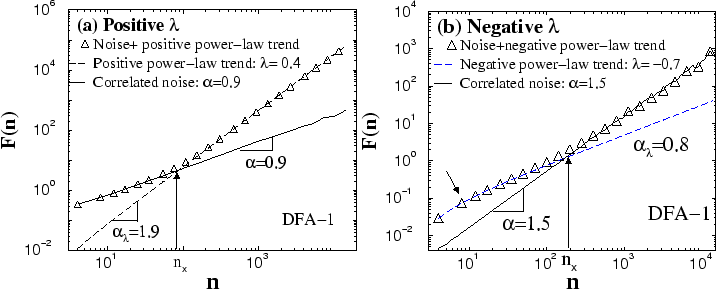
Figure 10: Crossover behavior of the rms fluctuation function $F_{\rm \eta P}(n)$ (circles) for correlated noise (of length $N_{max}=2^{17}$) with a superimposed power-law trend $u(i)=A_{\rm P} i^{\lambda}$. The rms fluctuation function $F_{\rm \eta}(n)$ for noise (solid line) and the rms fluctuation function $F_{\rm P}(n)$ (dash line) are also shown for comparison. DFA-1 method is used. (a) $F_{\rm \eta P}(n)$ for noise with correlation exponent $\alpha _{\lambda }=0.9$, and power-law trend with amplitude $A_{\rm P} = 1000/ {\left( N_{max}\right)^{0.4}}$ and positive power $\lambda =0.4$; (b) $F_{\rm \eta P}(n)$ for Brownian noise (integrated white noise, $\alpha _{\lambda }=1.5$), and power-law trend with amplitude $A_{\rm P} = 0.01/\left(N_{max}\right)^{-0.7}$ and negative power $\lambda =-0.7$. Note, that although in both cases there is a ``similar'' crossover behavior for $F_{\rm \eta P}(n)$, the results in (a) and (b) represent completely opposite situations: while in (a) the power-law trend with positive power $\lambda$ dominates the scaling of $F_{\rm \eta P}(n)$ at large scales, in (b) the power-law trend with negative power $\lambda$ dominates the scaling at small scales, with arrow we indicate in (b) a weak crossover in $F_{\rm P}(n)$ (dashed lines) at small scales for negative power $\lambda$.

In this section we study the effect of power-law trends on the scaling properties of noisy signals. We consider the case of correlated noise with superposed power-law trend $u(i)=A_{\rm P} i^{\lambda }$, when $A_{\rm P}$ is a positive constant, $i=1,...,N_{max}$, and $N_{max}$ is the length of the signal. We find that when the DFA-1 method is used, the rms fluctuation function $F_{\rm\eta P}(n)$ exhibits a crossover between two scaling regions [Fig. 10]. This behavior results from the fact that at different scales $n$, either the correlated noise or the power-law trend is dominant, and can be predicted by employing the superposition rule:

$$\left[F_{\rm\eta P}(n)\right]^2 = \left[F_{\rm\eta}(n)\right]^2 + \left[F_{\rm P}(n)\right]^2,$$ (18)

where $F_{\rm\eta }(n)$ and $F_{\rm P}(n)$ are the rms fluctuation function of noise and the power-law trend respectively, and $F_{\rm\eta P}(n)$ is the rms fluctuation function for the superposition of the noise and the power-law trend. Since the behavior of $F_{\rm\eta }(n)$ is known (Eq. (6) and Appendix 7.1), we can understand the features of $F_{\rm\eta P}(n)$, if we know how $F_{\rm P}(n)$ depends on the characteristics of the power-law trend. We note that the scaling behavior of $F_{\rm\eta P}(n)$ displayed in Fig. 10(a) is to some extent similar to the behavior of the rms fluctuation function $F_{\rm\eta L}(n)$ for correlated noise with a linear trend [Fig. 1] -- e.g. the noise is dominant at small scales $n$, while the trend is dominant at large scales. However, the behavior $F_{\rm P}(n)$ is more complex than that of $F_{\rm L}(n)$ for the linear trend, since the effective exponent $\alpha _{\lambda }$ for $F_{\rm P}(n)$ can depend on the power $\lambda$ of the power-law trend. In particular, for negative values of $\lambda$, $F_{\rm P}(n)$ can become dominated at small scales (Fig. 10(b)) while $F_{\rm\eta }(n)$ dominates at large scales -- a situation completely opposite of noise with linear trend (Fig. 1) or with power-law trend with positive values for the power $\lambda$. Moreover, $F_{\rm P}(n)$ can exhibit crossover behavior at small scales [Fig. 10(b)] for negative $\lambda$ which is not observed for positive $\lambda$. In addition $F_{\rm P}(n)$ depends on the order $\ell$ of the DFA method and the length $N_{max}$ of the signal. We discuss the scaling features of the power-law trends in the following three subsections.