A Brief Overview of Multifractal Time Series

Part 6: Multifractality of healthy human heart rate

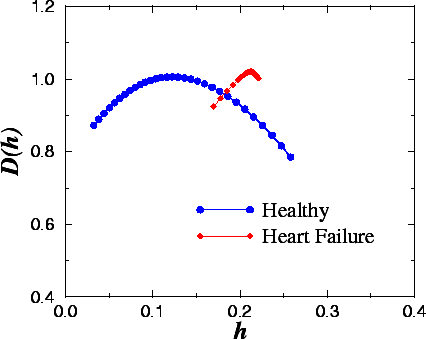
Multifractality has been uncovered in a number of fundamental physical and chemical processes (9). Recently, it was also reported that heart rate fluctuations of healthy individuals are multifractal (11). This finding posed new challenges to our understanding of heart rate regulation as most modeling of heart rate fluctuations over long time scales had concerned itself only with monofractal properties (12). For example, it appears that a major life-threatening condition, congestive heart failure, leads to a loss of multifractality (Fig. 8).


Local Holder exponent: Heart rate
Figure 8: Singularity spectra of the of heart rate signals for healthy and diseased hearts. Note the broad range of values of h with non-zero fractal dimensions for the healthy heart beat. This is indicative of multifractal dynamics. These dynamics are quite different from the ones responsible for the binomial multiplicative process as the two singularity spectra are quite different. For diseased patients, suffering from a life-threatening condition named congestive heart failure, we find a narrow range of values of h with non-zero fractal dimension. Even though this range is not really pulse-like, it is still likely that the dynamics are monofractal. The reason is that for finite signals there will always be some small error in the estimation of the value of h at a singularity. This error will lead to a small widening of the singularity spectrum (3).
More importantly, neither monofractal nor multifractal behaviors are accounted for by current understanding of physiological regulation based on homeostasis. Hence it would be beneficial, perhaps, to uncover how multifractality in the healthy heart dynamics arises. Two distinct possibilities can be considered. The first is that the observed multifractality is primarily a consequence of the response of neuroautonomic control mechanisms to activity-related fractal stimuli. If this were the case, then in the absence of such correlated inputs the heartbeat dynamics would not generate such a heterogeneous multifractal output. The second is that the neuroautonomic control mechanisms---in the presence of even weak external noise---endogenously generate multifractal dynamics.
Previous: Part 5: What one learns from the singularity spectra of multifractal signals