DFA-2 on noise with a linear trend

Figure 4: Comparison of the rms fluctuation function $F_{\rm \eta}(n)$ for noise with different types of correlations (lines) and $F_{\rm \eta L}(n)$ for the same noise with a linear trend of slope $A_{\rm L}=2^{-12}$ (symbols) for DFA-2. $F_{\rm \eta L}(n)=F_{\rm \eta}(n)$ because the integrated linear trend can be perfectly filtered out in DFA-2, thus $Y_{\rm L}(i) = 0$ from Eq.(3). We note, that to estimate accurately the correlation exponents one has to choose an optimal range of scales $n$, where $F(n)$ is fitted. For details see Appendix 7.1

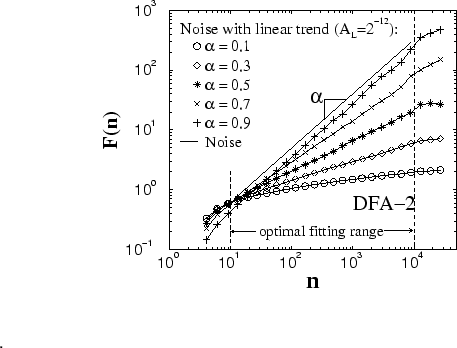Application of the DFA-2 method to noisy signals without any polynomial trends leads to scaling results identical to the scaling obtained from the DFA-1 method, with the exception of some vertical shift to lower values for the rms fluctuation function $F_{\rm\eta }(n)$ [see Appendix 7.1]. However, for signals which are a superposition of correlated noise and a linear trend, in contrast to the DFA-1 results presented in Fig. 1, $F_{\rm\eta L}(n)$ obtained from DFA exhibits no crossovers, and is exactly equal to the rms fluctuation function $F_{\rm\eta }(n)$ obtained from DFA-2 for correlated noise without trend (see Fig. 4). These results indicate that a linear trend has no effect on the scaling obtained from DFA-2. The reason for this is that by design the DFA-2 method filters out linear trends, i.e. $Y_{\rm L}(i) = 0$ (Eq.( 3)) and thus $F_{\rm\eta L}(n)=F_{\rm\eta }(n)$ due to the superposition rule (Eq. (8)). For the same reason, polynomial trends of order lower than $\ell$ superimposed on correlated noise will have no effect on the scaling properties of the noise when DFA-$\ell$ is applied. Therefore, our results confirm that the DFA method is a reliable tool to accurately quantify correlations in noisy signals embedded in polynomial trends. Moreover, the reported scaling and crossover features of $F(n)$ can be used to determine the order of polynomial trends present in the data.