DFA-1 on sinusoidal trend

Given a sinusoidal trend $u(i)= A_{\rm S} \sin \left(2\pi i/T\right)$ ( $i=1,...,N_{max}$), where $A_{\rm S}$ is the amplitude of the signal and $T$ is the period, we find that the rms fluctuation function $F_{\rm S}(n)$ does not depend on the length of the signal $N_{max}$, and has the same shape for different amplitudes and different periods [Fig. 5]. We find a crossover at scale corresponding to the period of the sinusoidal trend

$$n_{2\times} \approx T,$$ (11)

and does not depend on the amplitude $A_{\rm S}$. We call this crossover $n_{2\times }$ for convenience, as we will see later. For $n~<~ n_{2\times}$, the rms fluctuation $F_{\rm S}(n)$ exhibits an apparent scaling with the same exponent as $F_{\rm L}(n)$ for the linear trend [see Eq. (7)]:

$$F_{\rm S}(n) = k_1 \frac{A_{\rm S}}{T} n^{\alpha_{\rm S}}$$ (12)

where $k_1$ is a constant independent of the length $N_{max}$, of the period $T$ and the amplitude $A_{\rm S}$ of the sinusoidal signal, and of the box size $n$. As for the linear trend [Eq.(7)], we obtain $\alpha_{\rm S}~=~2$ because at small scales (box size $n$) the sinusoidal function is dominated by a linear term. For $n > n_{2\times }$, due to the periodic property of the sinusoidal trend, $F_{\rm S}(n)$ is a constant independent of the scale $n$:

$$F_{\rm S}(n) = \frac{1}{2\sqrt{2} \pi} A_{\rm S} \cdot T.$$ (13)

The period $T$ and the amplitude $A_{\rm S}$ also affects the vertical shift of $F_{\rm S}(n)$ in both regions. We note that in Eqs.(12) and (13), $F_{\rm S}(n)$ is proportional to the amplitude $A_{\rm S}$, a behavior which is also observed for the linear trend [Eq. (7)].

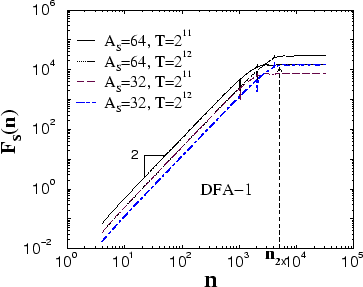
Figure 5: Root mean square fluctuation function $F_{\rm S}(n)$ for sinusoidal functions of length $N_{max}=2^{17}$ with different amplitude $A_{\rm S}$ and period $T$. All curves exhibit a crossover at $n_{2\times } \approx T/2$, with a slope $\alpha_{\rm S}=2$ for $n < n_{2\times }$, and a flat region for $n > n_{2\times }$. There are some spurious singularities at $n = j \frac{T}{2}$ ($j$ is a positive integer) shown by the spikes.