

Next: Evaluation
Up: ECG-based Indices of Physical
Previous: Correlates of physical activity
We may transform any sequence of interbeat intervals into an
instantaneous heart rate signal  , and derive a corresponding
trajectory in the 3-d space defined by
, and derive a corresponding
trajectory in the 3-d space defined by
 ,
,  ,
and
,
and  (Figure 1).
(Figure 1).
Figure 1:
Derivation of the activity index.
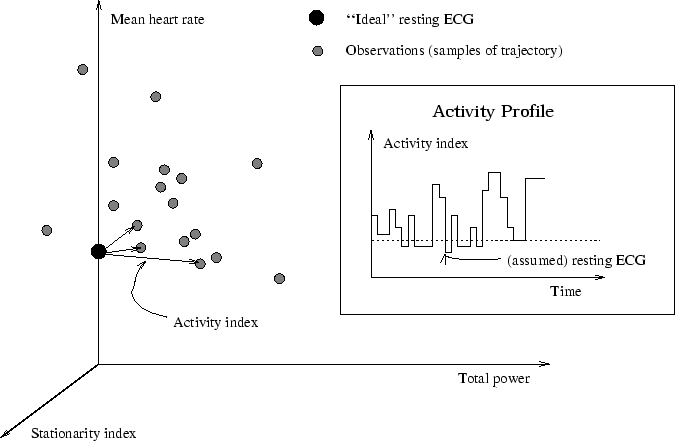 |
In general, one would expect to find that all three variables increase
with activity, and that the times of minimum activity would occur when
the trajectory most closely approaches the point indicated in Figure 1
as the ``ideal resting ECG''. This point,
 ,
does not coincide with the origin, since extremely low mean heart rate
measurements are likely to be erroneous; for this reason, it has been
placed at
,
does not coincide with the origin, since extremely low mean heart rate
measurements are likely to be erroneous; for this reason, it has been
placed at  beats per minute (bpm), with
beats per minute (bpm), with
 .
An activity index may be defined as the Euclidean distance of the
trajectory from the ``ideal resting ECG'':
.
An activity index may be defined as the Euclidean distance of the
trajectory from the ``ideal resting ECG'':
 |
(6) |
where the scaling constants may be determined empirically (for this
study,  ,
,  , and
, and  ), and
), and  is
a correction term for very low heart rate measurements:
is
a correction term for very low heart rate measurements:
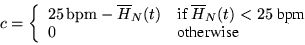 |
(7) |
In unusual cases,  may be large throughout a recording. This
can occur in the context of sustained atrial fibrillation, or if the
recording quality is uniformly poor, resulting in many detector
errors. In such cases, random fluctuations in
may be large throughout a recording. This
can occur in the context of sustained atrial fibrillation, or if the
recording quality is uniformly poor, resulting in many detector
errors. In such cases, random fluctuations in  might have a
dominant influence on
might have a
dominant influence on  . In HRV studies, such recordings are
typically excluded, so that this behavior is not usually a problem.
It may be avoided if necessary by placing an upper bound on
. In HRV studies, such recordings are
typically excluded, so that this behavior is not usually a problem.
It may be avoided if necessary by placing an upper bound on  before determining
before determining  .
.


Next: Evaluation
Up: ECG-based Indices of Physical
Previous: Correlates of physical activity
George B. Moody
2002-04-18
![]() , and derive a corresponding
trajectory in the 3-d space defined by
, and derive a corresponding
trajectory in the 3-d space defined by
![]() ,
, ![]() ,
and
,
and ![]() (Figure 1).
(Figure 1).
![]() may be large throughout a recording. This
can occur in the context of sustained atrial fibrillation, or if the
recording quality is uniformly poor, resulting in many detector
errors. In such cases, random fluctuations in
may be large throughout a recording. This
can occur in the context of sustained atrial fibrillation, or if the
recording quality is uniformly poor, resulting in many detector
errors. In such cases, random fluctuations in ![]() might have a
dominant influence on
might have a
dominant influence on ![]() . In HRV studies, such recordings are
typically excluded, so that this behavior is not usually a problem.
It may be avoided if necessary by placing an upper bound on
. In HRV studies, such recordings are
typically excluded, so that this behavior is not usually a problem.
It may be avoided if necessary by placing an upper bound on ![]() before determining
before determining ![]() .
.