Heart rate

Many definitions of heart rate are possible. This study makes use of both instantaneous heart rate, $H(t)$, and mean heart rate, $\overline{H}_{N}(t)$, as defined below.

Given the sequence of beat arrival times $R_{n}$, where $n$ denotes the beat number, a uniformly sampled instantaneous heart rate signal may be defined as

$$\textstyle{ H(t) = \frac{1}{2 k \Delta t} \left( \frac{R_{i... ... \frac{R_{j} - (t + {k \Delta t})}{R_{j} - R_{j-1}} \right) }$$ (1)

where $\Delta t$ is the desired sampling interval for $H(t)$, $k$ is a smoothing parameter, and $i$ and $j$ have been chosen such that

$$R_{i-1} \leq t - {k \Delta t} < R_{i} \atop R_{j-1} < t + {k \Delta t} \leq R_{j}$$ (2)

The middle term ($j-i$) in equation (1) thus represents one more than the number of complete interbeat intervals within a window of width $2 k \Delta t$ centered on $t$; the first term is the fraction of the previous interval within the window, and the last term is the fraction of the final interval that falls outside the window. From the instantaneous rate $H(t)$, we derive the mean heart rate:

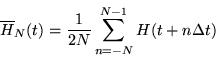$$\overline{H}_{N}(t) = \frac{1}{2N} \sum_{n=-N}^{N-1} H(t + n \Delta t)$$ (3)

In the present study, $\Delta t = 0.5$ seconds, $k = 1$, and $N = 300$ (i.e., mean heart rate is determined over a period of $2 N \Delta t = 5$ minutes).